In the realm of mathematics, the term “discriminant” holds a crucial position, especially when dealing with polynomial equations. This mathematical entity plays a pivotal role in determining various characteristics of these equations, influencing the nature of their solutions. In this comprehensive guide, we will delve deep into the concept of a discriminant, exploring its definition, formulas, and its impact on quadratic and cubic equations. Join us on this mathematical journey as we unlock the mysteries of discriminants.
✅ AI Essay Writer ✅ AI Detector ✅ Plagchecker ✅ Paraphraser
✅ Summarizer ✅ Citation Generator
Defining the Basics of Discriminant
A discriminant, in mathematics, is a function intricately tied to the coefficients of polynomial equations. At its core, it serves as a discriminative tool, providing insights into the nature of solutions that a polynomial equation possesses without explicitly finding those solutions. Essentially, it discriminates between various possibilities, distinguishing solutions as equal or unequal, real or nonreal. This mathematical marvel is commonly represented by the Greek letter Δ (Delta) or simply “D.”
To truly grasp the power of the discriminant, it’s essential to understand its formulas. In this section, we’ll break down the discriminant formulas for both quadratic and cubic equations.
Quadratic Discriminant Formula
In the context of quadratic equations, the discriminant formula takes center stage. For a quadratic equation in the form of ax^2 + bx + c = 0, the discriminant (Δ or D) is expressed as:
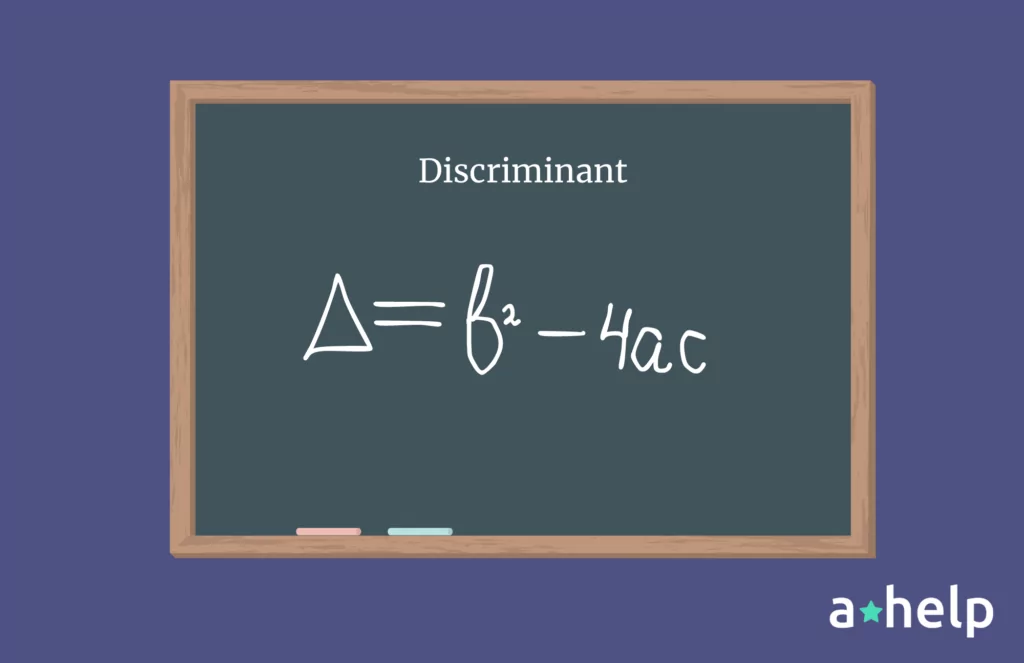
This fundamental formula lies at the heart of quadratic equations, serving as a key element in determining their nature.
Let’s put this formula to the test with an example. Consider the quadratic equation 2x^2 – 3x + 8 = 0. By comparing it to the standard form ax^2 + bx + c = 0, we identify a = 2, b = -3, and c = 8. Applying the discriminant formula, we calculate:
Δ or D = (-3)^2 – 4(2)(8) = 9 – 64 = -55
This result, Δ = -55, tells us a lot about the nature of the roots of the given quadratic equation.
Cubic Discriminant Formula
Moving beyond quadratic equations, cubic equations also have their own discriminant formula. For a cubic equation in the form of ax^3 + bx^2 + cx + d = 0, the discriminant (Δ or D) is expressed as:
Δ or D = b^2c^2 − 4ac^3 − 4b^3d − 27a^2d^2 + 18abcd
This formula provides valuable insights into the nature of the roots for cubic equations, albeit with a more complex mathematical structure.
Let’s consider the cubic equation x^3 – 3x + 2 = 0. By associating the equation with the standard form ax^3 + bx^2 + cx + d = 0, we find a = 1, b = 0, c = -3, and d = 2. Applying the discriminant formula, we calculate:
Δ or D = (0)^2(-3)^2 − 4(1)(-3)^3 − 4(0)^3(2) − 27(1)^2(2)^2 + 18(1)(0)(-3)(2) = 0 + 108 – 0 – 108 + 0 = 0
This result, Δ = 0, reveals crucial information about the nature of the roots in this cubic equation.
The Role of Discriminant in Determining Roots
The discriminant not only provides us with numerical values but also guides our understanding of the roots of polynomial equations. It helps us categorize the nature of these roots based on its value.
If Discriminant is Positive
When the discriminant (Δ or D) is greater than 0, it signifies that the quadratic equation has two distinct real roots. The expression inside the square root of the quadratic formula, b^2 – 4ac, is responsible for this categorization. A positive number under the square root always results in a real number. Therefore, when the discriminant is greater than 0, the equation boasts two distinct and real roots.
If Discriminant is Negative
On the other hand, when the discriminant (Δ or D) is less than 0, the quadratic equation boasts two distinct complex roots. The square root of a negative number leads to imaginary numbers. For example, when D < 0, the roots are given by x = ±√(-D)i. In this scenario, the equation possesses two distinct and complex roots, which are non-real.
If Discriminant is Equal to Zero
When the discriminant (Δ or D) equals 0, the quadratic equation possesses two equal real roots. In other words, there’s only one real root in the equation. This occurs because the square root of 0 is 0, reducing the equation to x = -b/2a, where there’s only one real number solution.
Graphs of Quadratic Functions
To further solidify our understanding, let’s explore how the discriminant affects the graphical representation of quadratic functions in each of the three cases mentioned earlier.
Case 1: Discriminant is Positive
When the discriminant is greater than 0, the quadratic equation has two distinct real roots. This is reflected in the graph, where the parabola intersects the x-axis at two distinct points. The roots are real and unequal, resulting in a parabola that opens upwards.
Case 2: Discriminant is Negative
In the case of a negative discriminant, the quadratic equation possesses two distinct complex roots. This scenario is depicted by a graph where the parabola does not intersect the x-axis. Instead, it hovers above or below it, representing complex roots.
Case 3: Discriminant is Equal to Zero
When the discriminant equals zero, the quadratic equation has two equal real roots. This translates to a graph where the parabola touches the x-axis at a single point, creating a tangent. In this case, there’s only one real root.
Important Notes on Discriminant
Before we conclude, let’s recap some essential notes about the discriminant:
- The discriminant of a quadratic equation ax^2 + bx + c = 0 is represented as Δ or D and calculated using the formula: Δ or D = b^2 − 4ac.
- A quadratic equation with a discriminant D can have two unequal real roots when D > 0, only one real root when D = 0, or no real roots when D < 0.
- The discriminant plays a vital role in determining the nature of roots, helping mathematicians understand the solutions to polynomial equations.
In the world of mathematics, the concept of a discriminant is nothing short of a mathematical compass. It guides us through the intricate terrain of polynomial equations, revealing the nature of their roots and solutions. Whether it’s a quadratic or cubic equation, the discriminant provides invaluable insights, helping us decode the mathematical mysteries hidden within. As you continue your journey in mathematics, remember that the discriminant is your ally, ready to navigate you through the fascinating world of polynomial equations.
Follow us on Reddit for more insights and updates.
More from Algebra Guides
Comments (0)
Welcome to A*Help comments!
We’re all about debate and discussion at A*Help.
We value the diverse opinions of users, so you may find points of view that you don’t agree with. And that’s cool. However, there are certain things we’re not OK with: attempts to manipulate our data in any way, for example, or the posting of discriminative, offensive, hateful, or disparaging material.