Geometry is a fascinating branch of mathematics that deals with the study of shapes, sizes, and spatial relationships. Within this realm, the midpoint formula plays a pivotal role in understanding the geometric properties of line segments. In this article, we will delve into the concept of the midpoint formula in geometry and explore its applications. Let’s embark on a journey to discover the equilibrium point in coordinate space.
✅ AI Essay Writer ✅ AI Detector ✅ Plagchecker ✅ Paraphraser
✅ Summarizer ✅ Citation Generator
At its core, the midpoint formula in geometry is a mathematical tool used to determine the exact center of a line segment defined by two endpoints. This formula finds the coordinates of the midpoint, denoted as “M,” which lies precisely between the two reference points. It is akin to finding the balance point of a seesaw.
The Midpoint Formula in Math
The formula itself is elegantly simple:
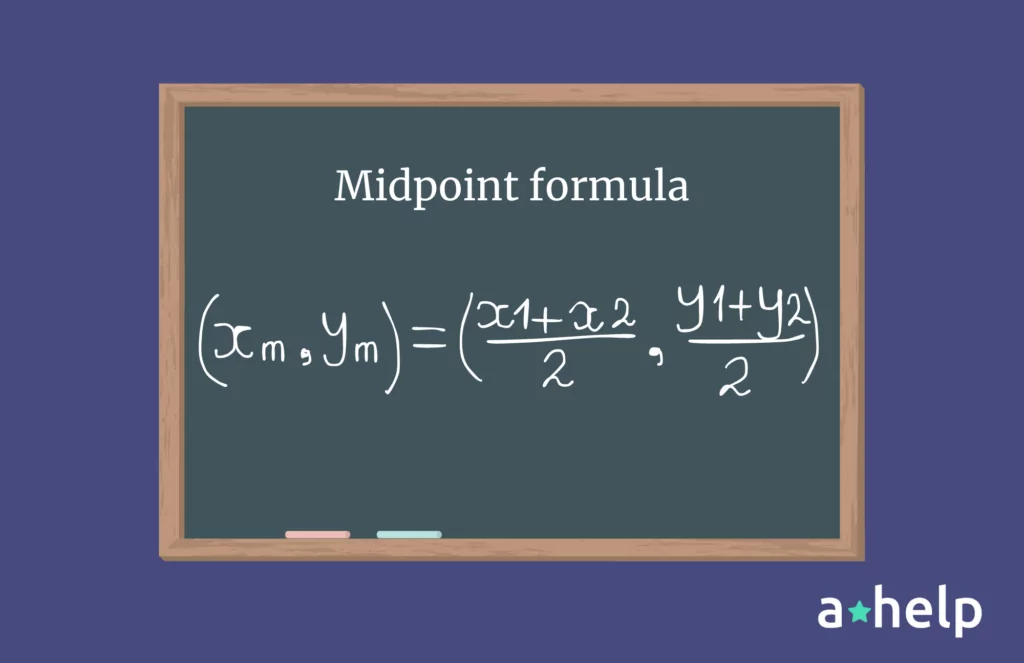
Here’s what these variables represent:
- M is the midpoint between the two endpoints, and (x3, y3) are its coordinates.
- (x1, y1) and (x2, y2) are the coordinates of the two endpoints.
This formula beautifully captures the essence of finding the middle ground in the vast coordinate plane. It ensures that we divide the line segment evenly along both the x and y axes.
Derivation of the Midpoint Formula
To grasp the underlying principles, let’s work through an example on a one-dimensional axis. Suppose we have two points, 5 and 9, on a number line. The midpoint is calculated as:
Midpoint = (5 + 9)/2 = 14/2 = 7
So, 7 serves as the midpoint between 5 and 9. Extending this idea to the coordinate plane, the formula is applied in a similar manner. It’s essentially the average of the x-coordinates for the x-coordinate of the midpoint and the average of the y-coordinates for the y-coordinate of the midpoint.
Applications of the Midpoint Formula
The midpoint formula finds its utility in various geometric scenarios, particularly when dealing with line segments. Let’s explore some of its applications.
1. Finding the Center of Circles
In geometry, circles are defined by their center points. The midpoint formula can be used to find the center of a circle when given the endpoints of its diameter.
Example: The diameter of a circle has endpoints, (2, -3) and (-6, 5). To find the coordinates of the center of the circle, we use the midpoint formula:
Center = ((2 + (-6))/2, (-3 + 5)/2) = (-4/2, 2/2) = (-2, 1)
Therefore, the center of the circle is (-2, 1).
2. Identifying the Midpoint of Line Segments
As its name suggests, the midpoint formula excels in identifying the midpoint of line segments. This information is crucial for various geometric calculations.
Example: Consider a line segment with endpoints (1, h) and (5, 7). To find the value of h if the midpoint is (3, -2), we use the formula:
Midpoint = (3, (h + 7)/2)
Equalizing this with the midpoint value (3, -2), we have (h + 7)/2 = -2, which leads to h = -11.
3. Exploring Geometric Properties
The midpoint formula helps us explore essential geometric properties, such as the balance of line segments and their division into equal parts.
Example: The endpoints of a line segment are (2, h) and (4, 7). To find the value of h if the midpoint is (3, -1), we apply the formula: Midpoint = (3, (h + 7)/2)
Solving for h, we find h = -9.
Midpoint Methods
In geometry, there are different methods to find the midpoint of a line segment, depending on the context and the nature of the segment. Let’s delve into two primary methods:
Method 1: Equal Division for Vertical or Horizontal Segments
If the line segment is vertical or horizontal, you can divide its length by 2 and count that value from any of the endpoints to reach the midpoint.
Example: The coordinates of points A and B are (-3, 2) and (1, 2) respectively. The length of the horizontal line is 4 units. Half of this length is 2 units. Moving 2 units from the point (-3, 2) gives us (-1, 2), which is the midpoint.
Method 2: The Midpoint Formula
For segments that are not necessarily vertical or horizontal, the midpoint formula provides a universal approach. It considers the coordinates of both endpoints and computes the midpoint, ensuring accuracy in any scenario.
Example: Using the midpoint formula, find the midpoint between points X(5, 3) and Y(7, 8).
Solution: Let M be the midpoint between X and Y. M = ((5 + 7)/2, (3 + 8)/2) = (6, 11/2). Therefore, the coordinates of the midpoint between X and Y are (6, 11/2).
Conclusion
In the realm of geometry, the midpoint formula is a valuable tool that empowers us to find balance and equilibrium in the vast coordinate plane. It plays a crucial role in identifying the center of circles, determining the midpoint of line segments, and exploring various geometric properties. Whether you’re dealing with horizontal, vertical, or diagonal segments, the midpoint formula stands as a reliable guide to uncover the heart of geometric figures. So, next time you encounter a line segment, remember the midpoint formula – your key to finding the perfect balance.
FAQ
Follow us on Reddit for more insights and updates.
More from Geometry Guides
Comments (0)
Welcome to A*Help comments!
We’re all about debate and discussion at A*Help.
We value the diverse opinions of users, so you may find points of view that you don’t agree with. And that’s cool. However, there are certain things we’re not OK with: attempts to manipulate our data in any way, for example, or the posting of discriminative, offensive, hateful, or disparaging material.