Right triangles are among the most commonly encountered triangles in geometry. Their unique nature—possessing one right angle—makes them particularly special. Among these, there are two types of right triangles which are deemed “special” due to the relationships between their angles and sides. These are the 30°-60°-90° triangle and the 45°-45°-90° triangle. In this article, we will explore these special right triangles, unravel the mystery behind their names, and learn some essential triangle formulas related to them.
✅ AI Essay Writer ✅ AI Detector ✅ Plagchecker ✅ Paraphraser
✅ Summarizer ✅ Citation Generator
Understanding the Basics of a Right Triangle
Before delving into our special right triangles, it’s vital to grasp the fundamental characteristics of a right triangle.
Definition: A right triangle is a triangle that has one angle measuring exactly 90°. This 90° angle is the defining feature of the right triangle.
The Hypotenuse: Every right triangle has a longest side, located opposite the right angle, known as the hypotenuse. It’s crucial to know the hypotenuse when working with triangle formulas, especially the Pythagorean theorem.
The 30°-60°-90° Triangle
One of the two special right triangles we are exploring is the 30°-60°-90° triangle. The name itself describes the three angles of the triangle.
Characteristics:
- One angle measures 30°.
- The second angle measures 60°.
- The right angle measures 90°.
Triangle Formulas and Relationships: The sides of a 30°-60°-90° triangle are in a unique and consistent ratio. If the shortest side (opposite the 30° angle) is of length ‘a’. The side opposite the 60° angle (which is longer than the shortest side but shorter than the hypotenuse) will be a√3. The hypotenuse, opposite the 90° angle, will be 2a.
The 45°-45°-90° Triangle
This triangle is also known as an isosceles right triangle because it not only has a right angle but also has two congruent angles of 45°.
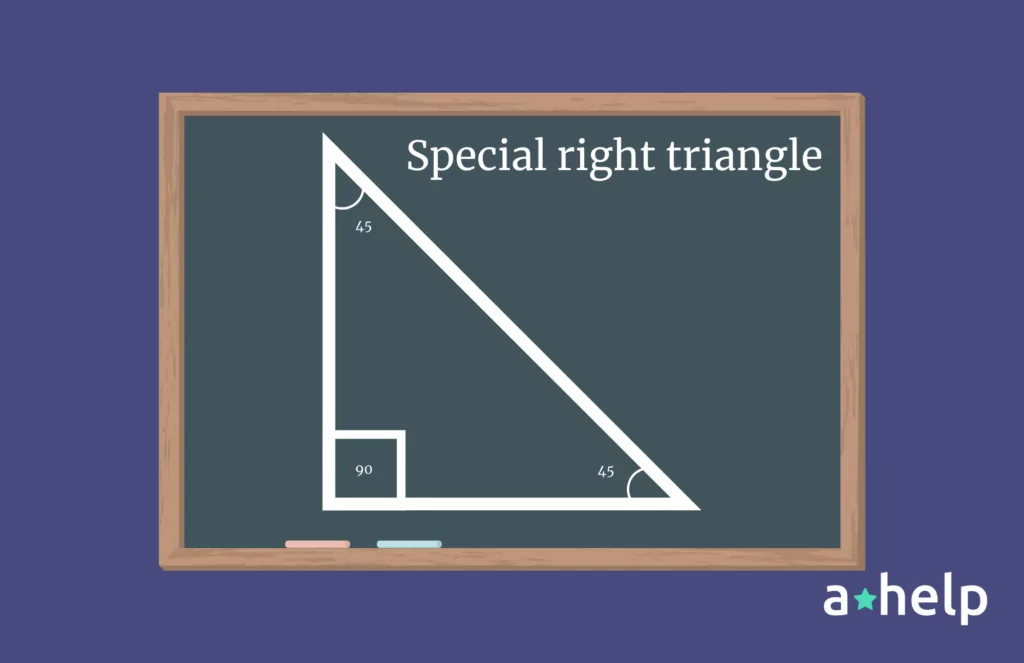
Characteristics:
- Two angles measure 45° each.
- The right angle measures 90°.
Triangle Formulas and Relationships: In a 45°-45°-90° triangle, the sides maintain a consistent relationship. If one leg (adjacent to a 45° angle) has a length of ‘b’. The other leg, which is opposite the other 45° angle, will also have a length of ‘b’ because the two legs are congruent. The hypotenuse will be b√2.
Practical Applications of Special Right Triangles
Why are these triangles deemed “special”? Their consistent relationships between sides and angles make them highly predictable and valuable in various fields.
Architects often employ the ratios inherent in these triangles when designing roofs, ramps, and other structures to ensure stability and aesthetic appeal. Moreover, these triangles are foundational in the study of trigonometry. The triangle formulas and side relationships are used to derive many trigonometric identities and concepts. Finally, in your everyday life, whether determining the shortest distance between two points or estimating the height of a distant object, the principles of special right triangles frequently come to the rescue.
Special right triangles, namely the 30°-60°-90° and the 45°-45°-90° triangles, hold a unique place in the world of geometry. Their consistent internal relationships make them predictable, turning complex problems into solvable ones. By understanding the fundamental characteristics of a right triangle and mastering the triangle formulas of these special right triangles, one equips themselves with powerful tools for both academic and real-world applications.
FAQ
Follow us on Reddit for more insights and updates.
More from Geometry Guides
Comments (0)
Welcome to A*Help comments!
We’re all about debate and discussion at A*Help.
We value the diverse opinions of users, so you may find points of view that you don’t agree with. And that’s cool. However, there are certain things we’re not OK with: attempts to manipulate our data in any way, for example, or the posting of discriminative, offensive, hateful, or disparaging material.