What Is the Angular Momentum Formula?

Angular momentum is a fundamental concept in physics that describes the rotational motion of objects. It plays a crucial role in understanding the dynamics of spinning objects, from celestial bodies like galaxies to everyday phenomena like the rotation of wheels and gears. In this guide, we will delve into the angular momentum formula and explore its significance in the world of physics.

Angular Momentum Basics

Angular momentum, denoted as L, is a vector quantity that represents the rotational motion of an object. It is a measure of how fast an object is rotating and how much rotational inertia it possesses. To calculate angular momentum, we use the following formula:

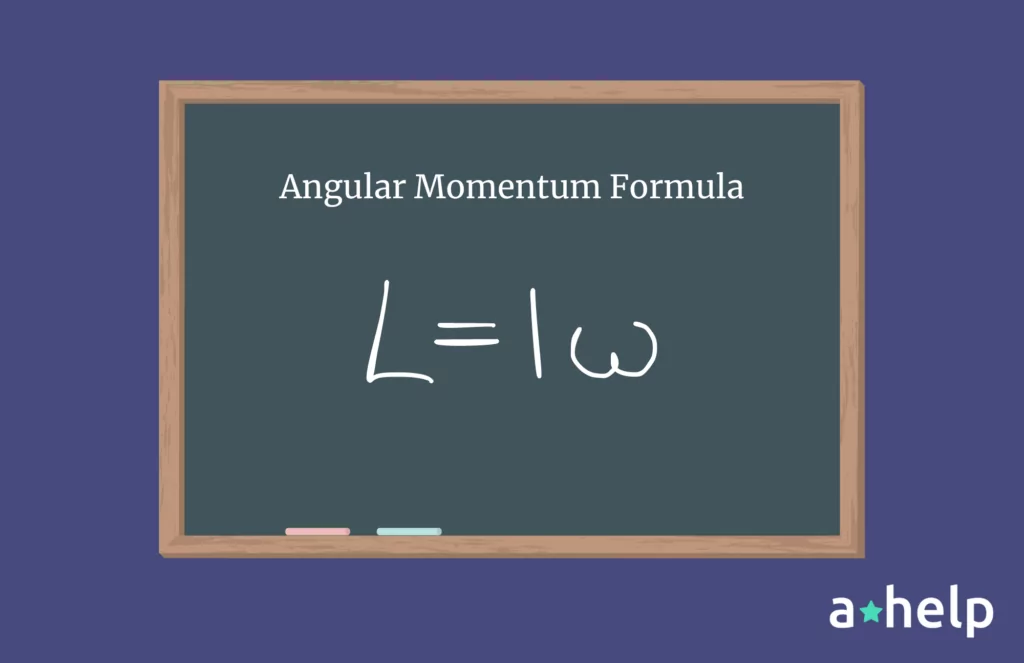
Here, L represents angular momentum, I is the moment of inertia of the object, and ω is its angular velocity. These terms are central to understanding angular momentum in depth.

Moment of Inertia (I)

Moment of inertia, often referred to as I, quantifies an object’s resistance to changes in its rotational motion. It depends on the distribution of mass within the object and its distance from the axis of rotation. The moment of inertia formula varies depending on the shape of the object. For instance, for a simple point particle, I equals mr^2, where m is the mass of the particle and r is the distance from the axis of rotation.

Angular Velocity (ω)

Angular velocity, represented as ω, measures how quickly an object rotates. It is defined as the change in angular displacement with respect to time. Angular velocity is expressed in radians per second (rad/s). For a rotating object, it is calculated as ω = Δθ/Δt, where Δθ is the change in angular displacement and Δt is the change in time.

Applications of Angular Momentum Formula

Angular momentum has diverse applications in various fields of physics, and the formula plays a pivotal role in understanding these phenomena.

Celestial Mechanics

Angular momentum is crucial in celestial mechanics, where it helps explain the motion of celestial bodies such as planets, stars, and galaxies. When considering celestial objects like planets orbiting the sun, their angular momentum is conserved due to the absence of external torques. This conservation of angular momentum keeps planets in stable orbits and influences phenomena like retrograde motion.

Rotational Mechanics

In the realm of rotational mechanics, the angular momentum formula is indispensable. For instance, when analyzing the rotation of a rigid body, understanding the distribution of mass within the body and its angular velocity allows us to calculate its angular momentum. This knowledge is vital in designing machines and engines with rotating parts, ensuring their stability and efficiency.

Conservation Laws

Angular momentum conservation is a fundamental principle in physics. According to this conservation law, the total angular momentum of an isolated system remains constant unless acted upon by external torques. This principle is encapsulated in the equation:

ΣL_initial = ΣL_final

It means that the sum of initial angular momenta is equal to the sum of final angular momenta, implying that angular momentum is conserved in isolated systems.

Practical Examples

Let’s apply the angular momentum formula to a practical example. Consider a spinning wheel, such as a bicycle wheel. In this scenario:

• Moment of Inertia (I): The moment of inertia of the wheel depends on its mass distribution and the distance of the mass from the axis of rotation. For a uniform circular disk, I = (1/2)mr^2, where m is the mass of the wheel and r is its radius.
• Angular Velocity (ω): The angular velocity of the spinning wheel is determined by how fast it rotates. For instance, if the wheel completes one full rotation in 2 seconds, ω = (2π rad) / (2 s) = π rad/s.

Now, we can calculate the angular momentum (L) of the spinning wheel using the formula:

L = Iω = [(1/2)mr^2] * π rad/s = (π/2)mr^2 rad/s

This result provides insight into the wheel’s angular momentum, which is crucial for maintaining balance while riding a bicycle.

Angular Momentum in Action

To further illustrate the concept of angular momentum, let’s explore its role in understanding the motion of celestial objects, particularly galaxies.

The Milky Way Galaxy

Galaxies, such as our own Milky Way, consist of billions of stars and other celestial objects. These galaxies exhibit complex rotational motion on a grand scale.

• Angular Momentum of the Galaxy: The total angular momentum of a galaxy is the vector sum of the angular momenta of all its constituent objects. This includes the angular momentum due to the spin and orbits of stars within the galaxy.
• Conservation of Angular Momentum: According to the conservation of angular momentum, as long as no external torques act on the galaxy, its total angular momentum remains constant. This principle helps explain the stability and motion of galaxies over cosmic timescales.
• Understanding Galaxy Evolution: By analyzing the angular momentum of galaxies and their components, astronomers gain insights into their formation and evolution. The distribution of angular momentum within galaxies informs us about their shape, rotation, and interactions with neighboring galaxies.

Conclusion

The angular momentum formula, L = Iω, serves as a fundamental tool for understanding rotational motion in the realm of physics. It allows us to quantify and analyze the rotational behavior of various objects, from spinning wheels to celestial bodies like galaxies. The conservation of angular momentum is a crucial principle that underpins our understanding of the dynamics of isolated systems, making angular momentum a cornerstone concept in the field of physics. Whether we’re exploring the universe or designing mechanical systems, a solid grasp of angular momentum and its formula is essential for gaining insights into the fascinating world of rotational motion.

FAQ

A*Help Insights - sign up for our Newsletter

Sign up

Thanks for signing up!

Something went wrong. Please try again later.

Follow us on Reddit for more insights and updates.